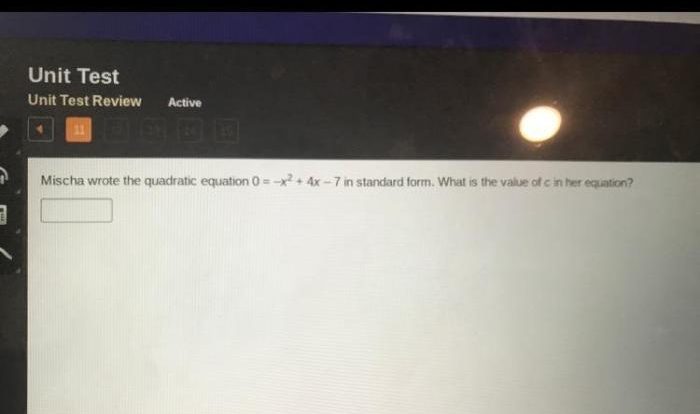
Prepare to unravel the mysteries of conic sections with our comprehensive Unit 9 Conic Sections Answer Key. This guide will illuminate the intricacies of circles, ellipses, parabolas, and hyperbolas, empowering you to conquer the complexities of these captivating curves.
Our answer key not only provides solutions but also delves into the fascinating world of conic sections, revealing their real-world applications and captivating properties. Join us on this enlightening journey and unlock the secrets of these geometric wonders.
Overview of Conic Sections: Unit 9 Conic Sections Answer Key
Conic sections are plane curves that result from the intersection of a plane with a double cone. They are classified into four main types: circles, ellipses, parabolas, and hyperbolas.
Circles
A circle is a plane curve that consists of all points that are equidistant from a given point, called the center. Circles are used in a variety of applications, such as wheels, gears, and compasses.
Ellipses
An ellipse is a plane curve that consists of all points that are the sum of the distances from two fixed points, called the foci, is constant. Ellipses are used in a variety of applications, such as orbits of planets and comets, and the shape of footballs.
Parabolas
A parabola is a plane curve that consists of all points that are equidistant from a fixed point, called the focus, and a fixed line, called the directrix. Parabolas are used in a variety of applications, such as the path of a projectile and the shape of satellite dishes.
Hyperbolas
A hyperbola is a plane curve that consists of all points that are the difference of the distances from two fixed points, called the foci, is constant. Hyperbolas are used in a variety of applications, such as the shape of orbits of comets and the design of bridges.
Properties of Conic Sections
Conic sections are curves that are formed by the intersection of a plane with a cone. There are three main types of conic sections: circles, ellipses, and hyperbolas. Each type of conic section has its own unique properties.
Eccentricity
Eccentricity is a measure of how much a conic section deviates from being a circle. It is defined as the ratio of the distance between the foci of the conic section to the length of its major axis. Eccentricity can range from 0 to 1. A conic section with an eccentricity of 0 is a circle, a conic section with an eccentricity between 0 and 1 is an ellipse, and a conic section with an eccentricity greater than 1 is a hyperbola.
Foci
The foci of a conic section are two points that are equidistant from every point on the conic section. The foci of a circle are the center of the circle. The foci of an ellipse are two points that are located on the major axis of the ellipse.
The foci of a hyperbola are two points that are located on the transverse axis of the hyperbola.
Asymptotes
Asymptotes are lines that the conic section approaches but never touches. A hyperbola has two asymptotes, which are the lines that the hyperbola approaches as it goes to infinity.
Table of Properties of Conic Sections
| Property | Circle | Ellipse | Hyperbola |
|---|---|---|---|
| Eccentricity | 0 | 0 < e < 1 | e > 1 |
| Foci | Center | On major axis | On transverse axis |
| Asymptotes | None | None | Two |
Equations of Conic Sections
Conic sections are curves that result from the intersection of a plane and a double cone. The standard equations for each type of conic section are as follows:
Circle
The standard equation of a circle with center (h, k) and radius r is:
(x
- h)^2 + (y
- k)^2 = r^2
Ellipse
The standard equation of an ellipse with center (h, k), semi-major axis length a, and semi-minor axis length b is:
(x
- h)^2 / a^2 + (y
- k)^2 / b^2 = 1
Parabola
The standard equation of a parabola with vertex (h, k) and axis of symmetry parallel to the y-axis is:
(x
- h)^2 = 4p(y
- k)
where p is the distance from the vertex to the focus.
Hyperbola, Unit 9 conic sections answer key
The standard equation of a hyperbola with center (h, k), transverse axis length 2a, and conjugate axis length 2b is:
(x
- h)^2 / a^2
- (y
- k)^2 / b^2 = 1
Converting Between Forms
It is sometimes necessary to convert between different forms of the equations for conic sections. For example, you may need to convert from the general form to the standard form or vice versa. The following steps can be used to convert between forms:
- Complete the square for the x-terms and y-terms.
- Factor out any common factors from the x-terms and y-terms.
- Identify the type of conic section based on the coefficients of the x-terms and y-terms.
- Rewrite the equation in the standard form for that type of conic section.
Graphing Conic Sections
Graphing conic sections involves plotting points that satisfy the equation of the conic section. Each type of conic section has its unique set of characteristics and steps for graphing.
Circle
To graph a circle with center (h, k) and radius r, follow these steps:
- Plot the center point (h, k).
- Use a compass to draw a circle with radius r, centered at (h, k).
Ellipse
To graph an ellipse with center (h, k), major axis length 2a, and minor axis length 2b, follow these steps:
- Plot the center point (h, k).
- Draw the major axis along the x-axis, with endpoints (h + a, k) and (h – a, k).
- Draw the minor axis along the y-axis, with endpoints (h, k + b) and (h, k – b).
- Plot points on the ellipse by using the parametric equations x = h + a cos(θ) and y = k + b sin(θ), where θ ranges from 0 to 2π.
Parabola
To graph a parabola with vertex (h, k) and axis of symmetry parallel to the y-axis, follow these steps:
- Plot the vertex point (h, k).
- Draw the axis of symmetry as a vertical line passing through (h, k).
- Plot points on the parabola by using the parametric equations x = h + at^2 and y = k + bt, where t ranges from -∞ to ∞.
Hyperbola, Unit 9 conic sections answer key
To graph a hyperbola with center (h, k), vertices (h ± a, k), and asymptotes y = (b/a)(x – h) ± k, follow these steps:
- Plot the center point (h, k).
- Draw the vertices (h ± a, k).
- Draw the asymptotes.
- Plot points on the hyperbola by using the parametric equations x = h + a sec(θ) and y = k + b tan(θ), where θ ranges from -π/2 to π/2.
Applications of Conic Sections
Conic sections are not just theoretical concepts; they have numerous practical applications in various fields. Their unique geometric properties make them essential tools for solving real-world problems.
Physics
*
-*Projectile Motion
The trajectory of a projectile, such as a thrown ball or a fired bullet, follows a parabolic path, which is a type of conic section. By understanding the principles of conic sections, physicists can calculate the velocity, height, and range of the projectile.
-*Orbits
The paths of celestial bodies, such as planets and satellites, are elliptical, which is another type of conic section. Kepler’s Laws of Planetary Motion describe the elliptical orbits of planets around the sun.
Engineering
*
-*Bridges
Suspension bridges use parabolic cables to support the roadway. The parabolic shape distributes the weight of the bridge evenly, ensuring structural integrity.
-*Satellite Dishes
Satellite dishes are designed using parabolic reflectors to focus radio waves from satellites. The parabolic shape reflects the waves in a specific direction, enabling communication with satellites.
Architecture
*
-*Arches
Many architectural structures, such as bridges and cathedrals, feature arches that are shaped like parabolas. The parabolic shape provides stability and strength to the arch, allowing it to withstand significant loads.
-*Domes
Domes, such as the one on the Pantheon in Rome, are often constructed using elliptical shapes. The elliptical shape distributes the weight of the dome evenly, preventing collapse.
Quick FAQs
What are the key properties of conic sections?
Conic sections are characterized by their eccentricity, foci, and asymptotes. Eccentricity measures the deviation from a circle, foci are fixed points, and asymptotes are lines that the curve approaches but never touches.
How do I graph a conic section?
To graph a conic section, follow these steps: identify the type of conic section, determine its equation, find the center and vertices, and sketch the curve using the appropriate techniques.
What are some real-world applications of conic sections?
Conic sections find applications in fields such as physics (projectile motion), engineering (bridge design), and architecture (dome construction). They also play a crucial role in astronomy, describing the orbits of celestial bodies.